Comparing different height-diameter models of Pinus sylvestris var. mongolica in sandy land
-
摘要:目的
比较不同树高(H)–胸径(D)模型精度，确定适合章古台地区樟子松Pinus sylvestris var. mongolica的H-D模型。
方法以Sibbesen模型为基础模型，将优势木平均高(HT)、胸高断面积(AB)和平方平均胸径(DQM) 3个林分变量以不同组合加入基础模型中，分别建立了H-D的基础模型(1个)和广义模型(3个)及对应的基础混合模型(1个)和广义混合模型(3个)。对固定效应模型平均水平预测(FPA)、混合模型的总体平均响应预测(MPA)和主体响应预测(MPS)的精度进行比较。对混合模型在使用随机抽取样本木和抽取平均木(胸径接近平均值的样本)2种抽样方案计算随机参数时分析MPS精度与样本数量的关系。
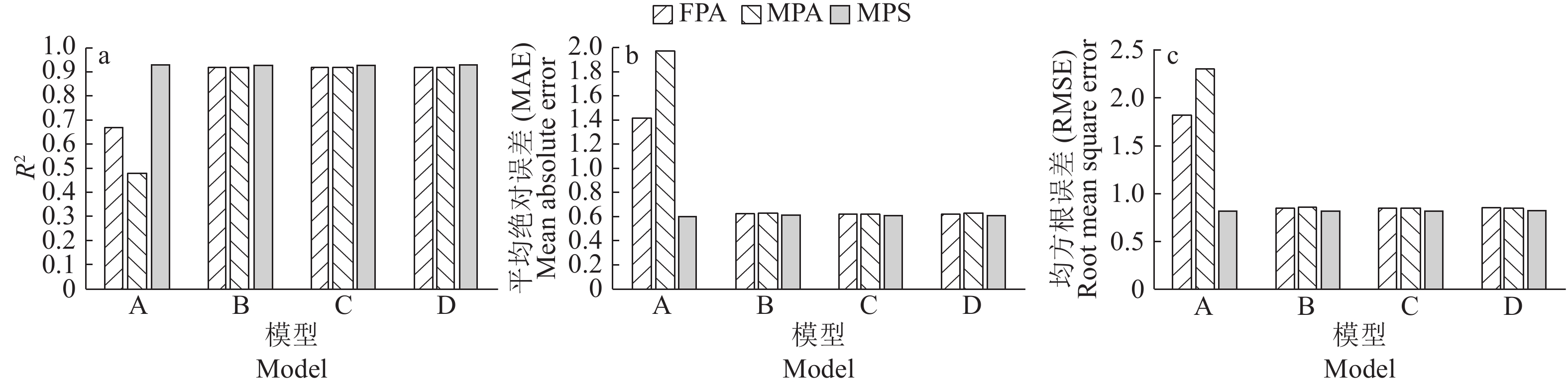
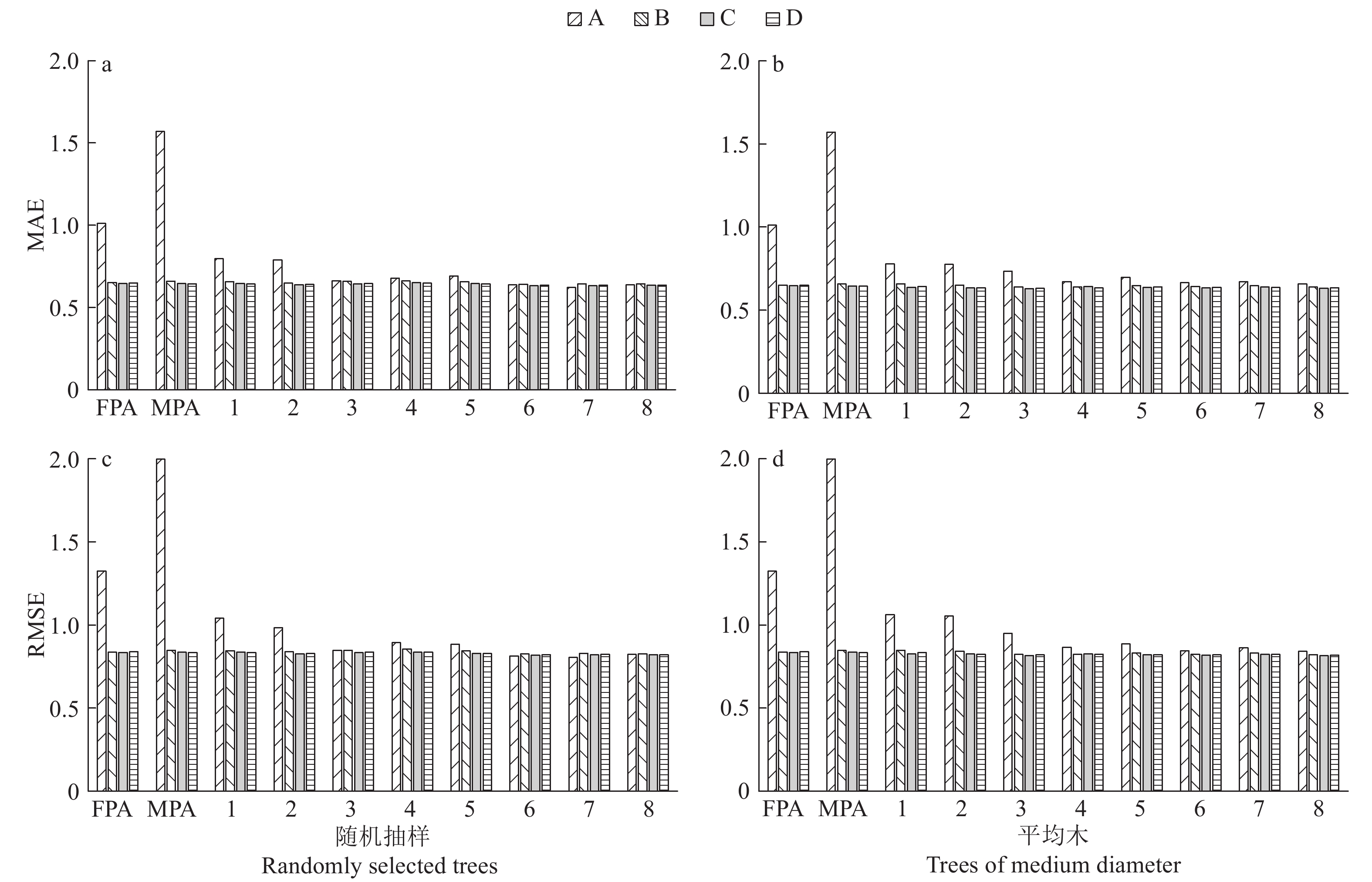
结果表征樟子松H-D关系的4种固定效应模型中，含HT和AB的广义模型拟合精度最高，Akaike信息量准则(AIC)=2 167.7，Bayesian信息量准则(BIC)=2 196.3。相同预测变量的各模型预测精度均表现为：MPS>FPA>MPA，仅含预测变量D的模型的3种预测精度差异最大。广义模型、广义混合模型、基础混合模型预测精度差异不大。使用验证数据检验模型精度时，每块标准地中随机抽取3株样本木计算基础混合模型随机参数时，该模型精度提升最为明显，MAE和RMSE分别降低了57.97%和57.63%；而广义混合模型精度随抽取样本木数量的增多未出现大的变化。
结论含有林分变量优势木平均高、胸高断面积的广义模型和基础混合模型均能较好地预测沙地樟子松人工林的单木树高。此外，利用混合模型预测树高时，推荐在标准地中随机抽取3株林木测量其树高，并依此来计算随机参数。
Abstract:ObjectiveTo compare the accuracy of different height(H)-diameter (D) models to determine the optimal models for Pinus sylvestris var. mongolica in Zhanggutai area.
MethodSibbesen model was used as the basic model. Dominant height (HT), stand basal area (AB), and quadratic mean diameter (DQM) with different combinations were added into Sibbesen model. We established one basic, three generalized, one basic mixed and three generalized mixed H-D models. The accuracies of population-averaged prediction (FPA) of fixed effects models, and mean response prediction (MPA) and specific-plot prediction (MPS) of mixed effects models were compared. For mixed models, two sampling designs, random sampling and medium-diameter tree sampling were used for random parameters estimation, and the relationship between MPS accuracy and sample size was analyzed.
ResultIn four fixed H-D models, the generalized model with HT and AB has the highest prediction precision. Akaike’s information criterion (AIC) is 2 167.7. Bayesian information criterion (BIC) is 2 196.3. Models with the same predictor variables have precision in order of MPS> FPA>MPA, and models withD as the only variable have the largest variation among three types of prediction. There are little difference in prediction accuracy among generalized models, generalized mixed models and basic mixed model. Using three randomly selected sample trees per plot to estimate random parameters of basic mixed model results in the highest model precision based on the validation data, and MAE and RMSE decrease by 57.97% and 57.63% respectively. The accuracies of generalized mixed models do not change significantly with the increase of sample size.
ConclusionBoth generalized model including HT and AB and basic mixed model can well predict tree height for P. sylvestris var. mongolica. We recommend to randomly select three sample trees per plot measuring tree heights for parameters estimation of mixed models, and calculating random parameters.
-
-
![]()
图 2 基于建模数据计算所得不同模型的不同预测类型指标统计
A：式(6)和(10)；B：式(7)和(11)；C：式(8)和(12)；D：式(9)和(13)
Figure 2. Indices calculated based on model fitting data for different types of predictions in different models
A: Eq.(6) and (10); B: Eq.(7) and (11); C: Eq.(8) and (12); D: Eq.(9) and (13)
![]()
图 3 不同抽样方案下各模型平均绝对误差(MAE)和均方根误差(RMSE)随样本木数量变化情况
FPA中，A~D依次为式(6)~(9)；MPA及1~8抽样中，A~D依次为式(10)~(13)
Figure 3. Relationship between mean absolute error (MAE), root mean square error (RMSE) and number of sample trees based on different sampling designs
FPA: A-D are eq.(6)-(9); MPA and different sampling design: A-D are eq. (10)-(13)
表 1 沙地樟子松标准地的林分与树木指标统计
Table 1 Summary statistics of stand and tree indices of Pinus sylvestris var. mongolica sample plot
变量1)
Variable总数据 Total data 建模数据 Modeling data 检验数据 Validation data $\overline x \pm {\rm{SE}} $ 最小值
Min.最大值
Max.$\overline x \pm {\rm{SE}} $ 最小值
Min.最大值
Max.$\overline x \pm {\rm{SE}} $ 最小值
Min.最大值
Max.H/m 8.5±0.1 2.1 16.1 8.3±0.1 2.1 16.1 9.2±0.2 2.2 14.3 D/cm 14.6±0.2 5.0 29.1 14.3±0.2 15.0 29.1 15.8±0.3 5.1 28.6 Drange/cm 10.9±0.4 5.7 16.5 10.9±0.4 5.7 16.5 11.0±0.9 7.3 14.2 Dmax/cm 22.0±0.9 10.0 29.1 22.0±1.0 10.0 29.1 21.9±2.1 11.9 28.6 Dmin/cm 11.0±0.8 1.9 18.0 11.1±0.9 1.9 18.0 10.9±1.9 3.3 18.0 A/a 36±2 13 62 36±3 13 62 36±6 13 56 N/(trees·hm–2) 842±80 300 2 500 862±96 300 2 500 764±112 450 1 175 HT/m 9.7±0.5 3.2 13.6 9.7±0.6 3.2 13.6 10.0±1.1 4.1 12.7 AB/(m2·hm–2) 15.26±1.04 2.92 33.34 15.16±1.19 4.28 33.34 15.65±2.31 2.92 21.97 DQM/cm 16.7±0.9 7.0 24.0 16.6±1.0 7.0 23.9 16.8±1.9 7.7 21.9 1) Drange、Dmax 和Dmin 分别为样地内胸径的分布范围值、最大值和最小值；A：树龄；N：林分密度；HT：优势木平均高；AB：胸高断面积；DQM：平方平均胸径
1) Drange, Dmax and Dminare the distribution range value, maximum and minimum of DBH in the plot; A: Age of stand; N: Stand density; HT: Dominant height; AB: Stand basal area; DQM: Quadratic mean diameter
下载: 导出CSV
表 2 基础和广义模型各参数估计结果及统计量
Table 2 Parameter estimates and evaluation statistics of basic and generalized models
公式 Formula β1 β2 β3 β4 β5 β6 σ2 AIC BIC LL (6) 14.242 3 –27.039 5 1.784 9 3.305 1 3 498.3 3 517.4 –1 745.2 (7) –0.105 1 –5.428 3 1.442 1 1.073 6 0.724 7 2 184.8 2 208.6 –1 087.4 (8) 0.190 2 –4.472 6 1.217 7 1.112 6 0.055 7 0.709 8 2 167.7 2 196.3 –1 077.9 (9) 0.404 3 –4.646 1.222 3 1.177 7 0.045 9 –0.034 5 0.709 3 2 168.2 2 201.5 –1 077.1
下载: 导出CSV
表 3 混合模型参数估计及统计量
Table 3 Parameter estimates and evaluation statistics of mixed model
公式 Formula β1 β2 β3 β4 β5 β6 σi2 σ2 AIC BIC LL (10) 10.258 1 –7.630 1 1.565 7 9.617 6 0.682 2 284.3 2 308.2 –1 137.2 (11) 0.211 3 –6.414 4 1.491 4 1.046 9 7.98×10−3 0.681 2 163.3 2 191.9 –1 075.7 (12) 0.248 3 –5.571 8 1.378 5 1.030 0 0.043 0 5.81×10−3 0.680 2 158.2 2 191.6 –1 072.1 (13) 0.375 3 –5.693 8 1.380 3 1.085 9 0.034 9 –0.029 1 5.65×10−3 0.680 2 159.3 2 197.4 –1 071.6
下载: 导出CSV
-
[1] SHARMA M. Comparing height-diameter relationships of boreal tree species grown in plantations and natural stands[J]. For Sci, 2016, 62(1): 70-77.
[2] HUANG S M, TITUS S J. An age-independent individual tree height prediction model for boreal spruce-aspen stands in Alberta[J]. Can J For Res, 1994, 24(7): 1295-1301. doi: 10.1139/x94-169
[3] 陈义刚, 谢正生, 张祥生, 等. 粤北低山丘陵地区小红栲生长过程分析[J]. 华南农业大学学报, 1994, 15(2): 124-128. [4] CORRAL-RIVAS S, ÁLVAREZ-GONZÁLEZ J G, CRECENTE-CAMPO F, et al. Local and generalized height-diameter models with random parameters for mixed, uneven-aged forests in Northwestern Durango, Mexico[J]. For Ecosyst, 2014, 1(1): 6. doi: 10.1186/2197-5620-1-6
[5] 臧颢, 雷相东, 张会儒, 等. 红松树高–胸径的非线性混合效应模型研究[J]. 北京林业大学学报, 2016, 38(6): 8-16. [6] 樊伟, 许崇华, 崔珺, 等. 基于混合效应的大别山地区杉木树高–胸径模型比较[J]. 应用生态学报, 2017, 28(9): 2831-2839. [7] HUANG S M, WIENS D P, YANG Y Q, et al. Assessing the impacts of species composition, top height and density on individual tree height prediction of quaking aspen in boreal mixed woods[J]. For Ecol Manage, 2009, 258(7): 1235-1247. doi: 10.1016/j.foreco.2009.06.017
[8] LEI X D, PENG C H, WANG H Y, et al. Individual height-diameter models for young black spruce (Picea mariana) and jack pine (Pinus banksiana) plantations in New Brunswick, Canada[J]. For Chron, 2009, 85(1): 43-56. doi: 10.5558/tfc85043-1
[9] ZEIDE B, VANDERSCHAAF C. The effect of density on the height-diameter relationship[C]// OUTCALT K W. Proceedings of the 11th biennial southern silvicultural research conference. Knoxville: General Technical Report SRS-48, 2002: 463-466.
[10] VAN LAAR A, ACKA A. Forest Mensuration[M]. Heidelberg: Springer, 2007: 383.
[11] SHARMA M, ZHANG S Y. Height-diameter models using stand characteristics for Pinus banksiana and Picea mariana[J]. Scand J For Res, 2004, 19(5): 442-451. doi: 10.1080/02827580410030163
[12] 符利勇, 张会儒, 唐守正. 基于非线性混合模型的杉木优势木平均高[J]. 林业科学, 2012, 48(7): 68-71. [13] 王冬至, 张冬燕, 张志东, 等. 基于非线性混合模型的针阔混交林树高与胸径关系[J]. 林业科学, 2016, 52(1): 30-36. doi: 10.3969/j.issn.1006-1126.2016.01.006 [14] CALAMA R, MONTERO G. Interregional nonlinear height-diameter model with random coefficients for stone pine in Spain[J]. Can J For Res, 2004, 34(1): 150-163. doi: 10.1139/x03-199
[15] 符利勇, 孙华, 张会儒, 等. 不同郁闭度下胸高直径对杉木冠幅特征因子的影响[J]. 生态学报, 2013, 33(8): 2434-2443. [16] SHARMA R P, BREIDENBACH J. Modeling height-diameter relationships for Norway spruce, Scots pine, and downy birch using Norwegian national forest inventory data[J]. For Sci Technol, 2015, 11(1): 44-53.
[17] ZANG H, LEI X, ZENG W. Height-diameter equations for larch plantations in northern and northeastern China: A comparison of the mixed-effects, quantile regression and generalized additive models[J]. Forestry, 2016, 89(4): 434-445. doi: 10.1093/forestry/cpw022
[18] 雷泽勇, 周晏平, 赵国军, 等. 竞争对辽宁西北部樟子松人工固沙林树高生长的影响[J]. 干旱区研究, 2018(1): 144-149. [19] 韩辉, 张学利, 党宏忠, 等. 基于树干液流通量的沙地樟子松合理林分密度的确定[J]. 林业科学研究, 2015, 28(6): 797-803. doi: 10.3969/j.issn.1001-1498.2015.06.006 [20] HUANG S, TITUS S J, WIENS D P. Comparison of nonlinear height-diameter functions for major Alberta tree species[J]. Can J For Res, 1992, 22(9): 1297-1304. doi: 10.1139/x92-172
[21] SIBBESEN E. Some new equations to describe phosphate sorption by soils[J]. J Soil Sci, 1981, 32(1): 67-74. doi: 10.1111/ejs.1981.32.issue-1
[22] YANG Y, HUANG S. Allometric modelling of crown width for white spruce by fixed- and mixed-effects models[J]. For Chron, 2017, 93(2): 138-147. doi: 10.5558/tfc2017-020
[23] MENG S X, HUANG S M, YANG Y Q, et al. Evaluation of population-averaged and subject-specific approaches for modeling the dominant or codominant height of lodgepole pine trees[J]. Can J For Res, 2009, 39(6): 1148-1158. doi: 10.1139/X09-039
计量
- 文章访问数:
- HTML全文浏览量: 0
- PDF下载量:
