Biomass growth models for individual tree of main indigenous broadleaf tree species in Guangdong Province
-
摘要:目的
选择广东主要乡土阔叶树种樟树Cinnamomum camphora、木荷Schima superba和枫香Liquidambar formosana为研究对象,建立3个树种的单木生物量生长模型,快速精确计量和监测森林碳汇造林项目的碳储量变化。
方法每个树种按10个径阶均匀分配伐倒90株样木(共270株),以样木的生物量数据为单木生物量,以立木年龄为自变量,分别建立不同起源(天然林和人工林) 3个树种的地上和地下4种方程生物量生长模型,并选择最优模型通过联立方程组总量控制法解决地上各组分(干材、树皮、树枝、树叶)的生长模型相容性问题。
结果天然林和人工林起源条件下,相同树种在同一生物量生长模型形式下生物量增长的上限值和最大增速年龄均有差异。各方程在相同起源和树种条件下所得的生物量上限和拐点年龄差异明显。估计地上生物量时,各树种最优方程形式不同。选择Logistic方程对3个树种地上各组分生物量联立方程组建立相容性生长模型,3个树种干材生物量方程的
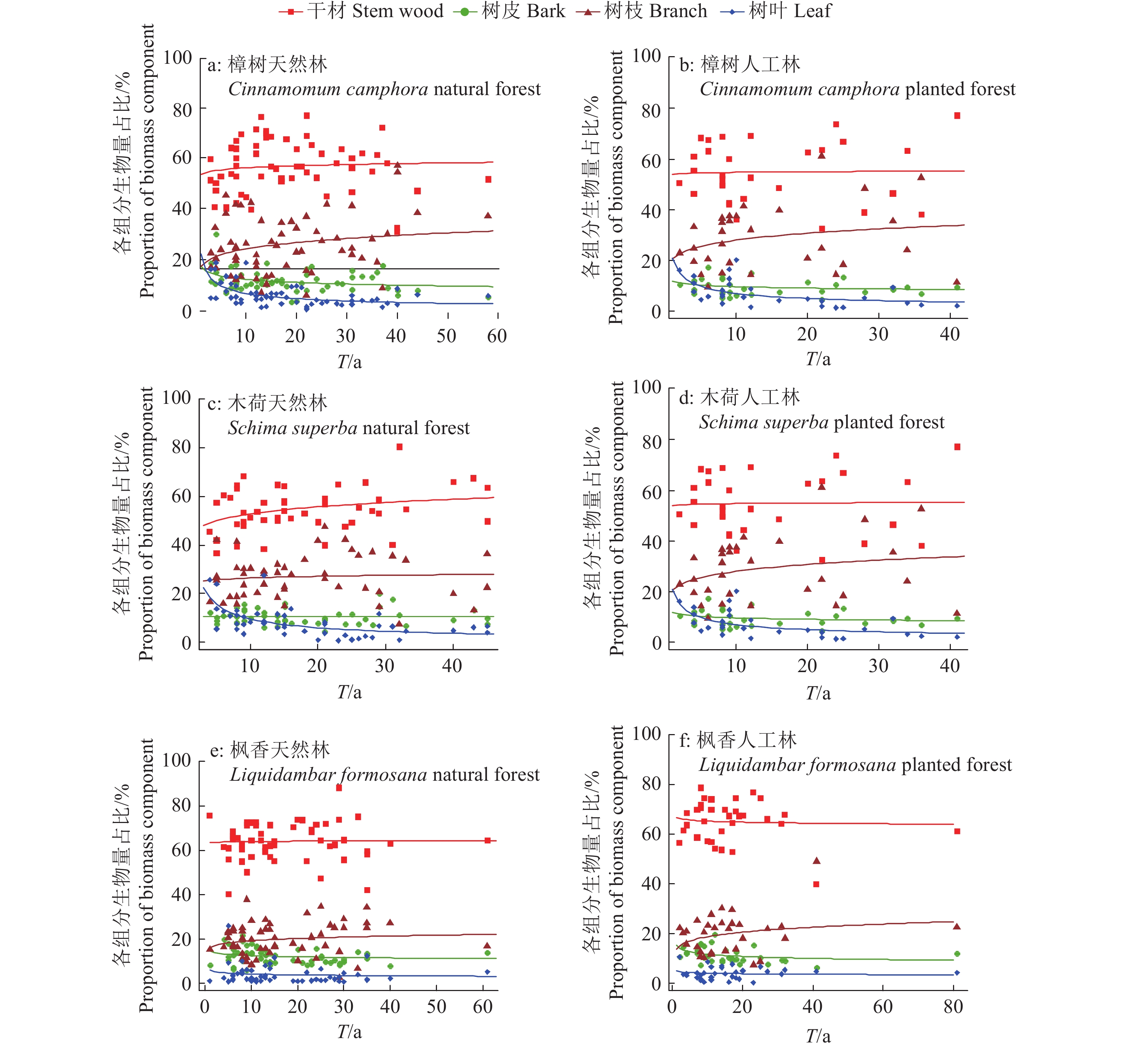
${{R}}_{\rm adj}^2$ 为0.560~0.768,平均预估误差(MPE)为3.05%~6.73%;树皮生物量方程的${{R}}_{\rm adj}^2$ 为0.552~0.866,MPE为2.02%~6.27%;树枝生物量方程的${{R}}_{\rm adj}^2$ 为0.309~0.706,MPE为3.01%~14.33%;树叶生物量方程的${{R}}_{\rm adj}^2$ 为0.495~0.767,MPE为4.16%~7.14%。结论比较4种模型的参数及评价指标可知,地上生物量生长最优模型为Logistic方程,地下生物量生长最优模型为Schumacher方程。地上各组分生物量在立木生长的周期中占地上总生物量的比例随着年龄的增长而不断变化。选择Logistic方程对3个树种地上各组分生物量联立方程组建立相容性生长模型,干材和树皮的生物量方程拟合效果相对于树枝和树叶更好。该模型主要适用于在已知年龄的人工碳汇造林的生物量估计;结合含碳系数,可预估未来一定时期内的碳储量及碳汇量。
Abstract:ObjectiveTo calculate quickly and precisely forest carbon sequestration in afforestation projects, we selected major broad-leaved tree species in Guangdong, including Cinnamomum camphora, Schima superba and Liquidambar formosana, and established biomass growth model of individual tree.
MethodAll 270 sample trees with 90 sample trees for each tree species were obtained according to 10 diameter classes during the process of modeling. We established four types of biomass growth models for aboveground and underground biomass of three tree species from different origins (natural forest or planted forest) using age as the independent variable. The compatibility issue among growth models of different aboveground components (stem wood, bark, branch, leaf) was solved using optimized models with a set of simultaneous equations and controlled total biomass.
ResultComparing trees from different origins including natural forest and planted forest, the biomass upper limits and ages of the maximum growth rate for the same species under the same biomass model were different. The biomass upper limits and ages of the maximum growth rate indicated by different equations for the same tree species under the same origin were largely different. When estimating the aboveground biomass, the optimal types of equations for different tree species were different. Logistic model was used to establish the compatibility model for the simultaneous equations of biomass for aboveground components of three tree species. The
${{R}}_{\rm adj}^2$ values from stem wood biomass equations of three species ranged from 0.560 to 0.768, and MPEs ranged from 3.05% to 6.73%. The${{R}}_{\rm adj}^2$ values from bark biomass equations ranged from 0.552 to 0.866, and the MPEs ranged from 2.02% to 6.27%. The${{R}}_{\rm adj}^2$ values from branch biomass equations ranged from 0.309 to 0.706, and the MPEs ranged from 3.01% to 14.33%. The${{R}}_{\rm adj}^2$ values from leaf biomass equations ranged from 0.495 to 0.767, and the MPEs ranged from 4.16% to 7.14%.ConclusionComparing the parameters and evaluation indexes of four models, the optimal model of aboveground biomass is the Logistic model and the optimal model of underground biomass is the Schumacher model. The proportion of each aboveground component in total aboveground biomass constantly changes with age during the growth process. The compatibility model for the simultaneous equations of biomass for aboveground components of three tree species is established using Logistic model, and the fitting effects of biomass models for stem wood and bark biomass are better than those for branch and leaf. These biomass models could estimate forest carbon combined with carbon coefficient in planted forest for known age in a certain period.
-
续表 1 Continued table 1 树种
Species起源
Origin变量
Variable最小值
Min.最大值
Max.平均值
Mean标准差
SD样木株数
No. of trees樟树
Cinnamomum camphora人工林
Planted forest干皮生物量/kg Bark biomass 0.09 65.24 8.99 14.26 30 树枝生物量/kg Branch biomass 0.10 553.75 46.93 111.00 30 树叶生物量/kg Leaf biomass 0.06 22.84 4.30 6.16 30 地上生物量/kg Aboveground biomass 0.87 948.38 117.11 206.06 30 地下生物量/kg Underground biomass 0.38 330.29 48.19 96.82 11 木荷 天然林 DBH/cm 1.70 51.50 15.73 12.05 47 Schima Natural forest H/m 3.02 23.10 10.25 4.78 47 superba T 4.00 45.00 18.21 11.10 47 干材生物量/kg Stem wood biomass 0.28 570.65 85.49 132.23 47 干皮生物量/kg Bark biomass 0.07 89.02 14.87 22.24 47 树枝生物量/kg Branch biomass 0.10 267.12 40.25 63.80 47 树叶生物量/kg Leaf biomass 0.14 35.83 6.76 8.63 47 地上生物量/kg Aboveground biomass 0.61 897.40 147.36 211.59 47 地下生物量/kg Underground biomass 0.43 173.30 30.41 42.59 20 人工林 DBH/cm 2.10 38.90 12.87 9.10 43 Planted forest H/m 2.50 18.70 10.15 4.21 43 T 4.00 31.00 15.14 7.83 43 干材生物量/kg Stem wood biomass 0.30 360.66 51.43 75.61 43 干皮生物量/kg Bark biomass 0.06 65.92 10.19 15.54 43 树枝生物量/kg Branch biomass 0.25 371.04 31.95 68.52 43 树叶生物量/kg Leaf biomass 0.17 45.70 4.57 8.69 43 地上生物量/kg Aboveground biomass 0.89 834.05 98.13 164.15 43 地下生物量/kg Underground biomass 0.20 670.84 46.40 148.22 20 枫香 天然林
DBH/cm 1.80 43.50 14.64 10.28 57 Liquidambar Natural forest H/m 3.00 26.60 11.78 5.13 57 formosana T 1.00 61.00 17.02 11.37 57 干材生物量/kg Stem wood biomass 0.21 569.88 80.48 120.15 57 干皮生物量/kg Bark biomass 0.06 121.59 13.61 20.26 57 树枝生物量/kg Branch biomass 0.06 147.26 25.74 38.78 57 树叶生物量/kg Leaf biomass 0.01 46.38 4.13 8.87 57 地上生物量/kg Aboveground biomass 0.35 884.61 123.96 177.36 57 地下生物量/kg Underground biomass 0.20 161.38 37.77 45.68 25 人工林 DBH/cm 2.20 39.60 14.09 11.14 33 Planted forest H/m 3.20 21.00 11.54 5.66 33 T 2.00 81.00 16.73 14.62 33 干材生物量/kg Stem wood biomass 0.45 426.45 83.16 140.32 33 干皮生物量/kg Bark biomass 0.09 78.97 12.31 20.89 33 树枝生物量/kg Branch biomass 0.15 468.70 37.31 89.96 33 树叶生物量/kg Leaf biomass 0.02 46.04 5.39 10.77 33 地上生物量/kg Aboveground biomass 0.71 955.54 138.17 249.06 33 地下生物量/kg Underground biomass 0.31 574.90 47.05 146.39 15 表 1 3个树种生物量建模数据统计
Table 1 Statistics of biomass modeling data for three tree species
树种
Species起源
Origin变量
Variable最小值
Min.最大值
Max.平均值
Mean标准差
SD样木株数
No. of trees樟树
Cinnamomum camphora天然林
Natural forest胸径/cm Diameter at breast height (DBH) 1.90 41.00 14.62 10.12 60 树高/m Height (H) 1.86 16.60 9.58 3.70 60 年龄 Tree age (T) 3.00 58.00 18.41 12.13 60 干材生物量/kg Stem wood biomass 0.22 519.66 65.07 105.70 60 干皮生物量/kg Bark biomass 0.05 75.54 11.28 16.87 60 树枝生物量/kg Branch biomass 0.20 499.48 47.80 110.06 60 树叶生物量/kg Leaf biomass 0.03 89.77 6.47 14.70 60 地上生物量/kg Aboveground biomass 0.43 1 022.66 129.75 236.57 60 地下生物量/kg Underground biomass 0.14 304.55 45.63 80.08 29 人工林
Planted forestDBH/cm 2.00 40.00 14.32 11.11 30 H/m 1.70 17.60 8.42 4.03 30 T 2.00 51.00 14.77 10.90 30 干材生物量/kg Stem wood biomass 0.44 360.82 56.02 87.61 30 表 2 以立木年龄为变量的生物量生长模型参数1)
Table 2 Parameters of biomass growth models with tree age as the independent variable
树种
Species起源
Origin组分
ComponentShumacher 模型
Shumacher modelChapman-Richards 模型
Chapman-Richards modelLogistics 模型
Logistics model${\alpha _1}$ ${\alpha _2}$ ${\beta _1}$ ${\beta _2}$ ${\beta _3}$ ${\lambda _1}$ ${\lambda _2}$ ${\lambda _3}$ 樟树Cinnamomum camphora 天然林
Natural
forest地上
Aboveground5.007×103 9.224×101 1.296×104 8.985×10–3 2.770 1.205×103 5.258 0.124 地下
Underground1.349×104 1.686×102 − − − − − − 人工林
Planted
forest地上
Aboveground1.519×103 4.445×101 8.071×102 5.545×10–2 4.051 5.706×102 4.538 0.168 地下
Underground5.180×102 3.209×101 − − − 2.335×102 7.419 0.407 木荷
Schima
superba天然林
Natural
forest地上
Aboveground1.412×103 4.351×101 5.292×102 1.136×10–1 11.340 4.735×102 5.241 0.220 地下
Underground1.938×102 3.329×101 − − − 8.874×101 7.096 0.310 人工林
Planted
forest地上
Aboveground6.030×103 7.935×101 − − − 1.183×103 5.792 0.176 地下
Underground2.155×109 4.495×102 − − − − − − 枫香
Liquidambar formosana天然林
Natural
forest地上
Aboveground2.051×103 5.690×101 1.726×103 2.462×10–2 2.737 9.198×102 4.089 0.109 地下
Underground3.334×102 4.008×101 − − − 9.478×101 16.460 0.739 人工林
Planted
forest地上
Aboveground1.391×103 3.832×101 7.807×102 1.079×10–1 12.460 7.820×102 5.265 0.189 地下
Underground1.777×107 4.239×102 − − − − − − 1) “−” 表示模型不收敛
1) “−” indicates non-convergence of the model表 3 以立木年龄为变量的生物量生长模型评价1)
Table 3 Evaluation of biomass growth models with tree age as the independent variable
树种
Species起源
Origin组分
Component模型
Model$R_{\rm adj}^2$ 平均偏差/kg
ME标准误/kg
SE平均预估
误差/%
MPE总相对
误差/%
TRE樟树
Cinnamomum camphora天然林
Natural
forest地上
AbovegroundSchumacher 0.607 17.31 149.49 3.84 –15.39 Chapman-Richards 0.621 3.62 146.97 3.78 –2.87 Logistic 0.634 4.82 144.41 3.71 –3.86 地下
UndergroundSchumacher 0.625 10.21 49.92 7.71 –28.81 Chapman-Richards − − − − − Logistic − − − − − 人工林
Planted
forest地上
AbovegroundSchumacher 0.551 3.87 142.91 8.31 –3.42 Chapman-Richards 0.553 1.20 142.50 8.28 –1.04 Logistic 0.558 –1.44 141.71 8.24 1.21 地下
UndergroundSchumacher 0.757 –2.59 52.69 21.88 5.09 Chapman-Richards − − − − − Logistic 0.814 1.58 46.15 19.16 –3.39 木荷
Schima
superba天然林
Natural
forest地上
AbovegroundSchumacher 0.582 –0.76 139.71 4.06 0.51 Chapman-Richards 0.588 3.63 138.81 4.03 –2.52 Logistic 0.584 –1.66 139.36 4.05 1.11 地下
UndergroundSchumacher 0.449 0.08 33.30 11.42 –0.28 Chapman-Richards − − − − − Logistic 0.536 2.75 30.57 10.48 –9.94 人工林
Planted
forest地上
AbovegroundSchumacher 0.603 6.78 104.63 5.00 –7.42 Chapman-Richards − − − − − Logistic 0.611 –1.18 103.58 4.95 1.19 地下
UndergroundSchumacher 0.978 10.03 22.69 5.10 –27.57 Chapman-Richards − − − − − Logistic − − − − − 枫香
Liquidambar formosana天然林
Natural
forest地上
AbovegroundSchumacher 0.809 6.10 78.81 2.23 –5.18 Chapman-Richards 0.815 –2.02 77.59 2.20 1.61 Logistic 0.794 –7.77 81.92 2.32 5.90 地下
UndergroundSchumacher 0.677 –0.30 27.06 5.91 0.80 Chapman-Richards − − − − − Logistic 0.725 3.44 24.95 5.44 –10.03 人工林
Planted
forest地上
AbovegroundSchumacher 0.635 –9.28 152.91 6.82 6.29 Chapman-Richards 0.695 4.64 139.83 6.24 –3.47 Logistic 0.706 –1.98 137.28 6.13 1.41 地下
UndergroundSchumacher 0.995 5.04 10.69 3.23 –12.01 Chapman-Richards − − − − − Logistic − − − − − 1) “−” 表示模型不收敛
1) “−” indicates non-convergence of the model表 4 以立木年龄为自变量的地上各组分生物量相容性生长模型参数1)
Table 4 Parameters of the compatibility growth models for biomass of different aboveground components with tree age as the independent variable ×10–2
树种 Species 起源 Origin a1 b1 a2 b2 a3 b3 樟树
Cinnamomum camphora天然林 Natural forest 7.26 23.13 55.10 8.17 10.41 –0.68 人工林 Planted forest 6.98 23.89 63.91 7.46 7.44 –1.39 木荷
Schima superba天然林 Natural forest 7.37 24.69 33.30 8.01 7.73 –1.17 人工林 Planted forest 8.73 26.30 38.57 17.05 9.81 –1.72 枫香
Liquidambar formosana天然林 Natural forest 7.28 24.50 24.66 7.46 5.47 –0.88 人工林 Planted forest 3.92 3.54 3.72 75.33 1.01 –258.50 1) a1、b1、a2、b2、a3、b3:模型参数
1) a1,b1,a2,b2,a3,b3: Parameters of models表 5 以立木年龄为自变量的地上各组分生物量相容性生长模型评价
Table 5 Evaluation of the compatibility growth models for biomass of different aboveground components with tree age as the independent variable
树种
Species起源
Origin组分
Component$R_{\rm adj}^2$ 平均偏差/kg
ME标准误/kg
SE平均预估误差/%
MPE总相对误差/%
TRE樟树
Cinnamomum camphora天然林
Natural
forest干材 Stem wood 0.622 2.44 67.92 3.48 0.04 树皮 Bark 0.552 1.12 11.80 3.49 0.11 树枝 Branch 0.519 1.15 80.51 5.72 0.03 树叶 Leaf 0.510 0.11 10.75 5.54 0.02 人工林
Planted
forest干材 Stem wood 0.768 –2.17 47.22 5.74 –0.04 树皮 Bark 0.730 0.13 8.27 6.27 0.01 树枝 Branch 0.309 0.43 100.61 14.33 0.01 树叶 Leaf 0.571 0.16 4.51 7.14 0.04 木荷
Schima superba天然林
Natural
forest干材 Stem wood 0.560 –3.37 93.84 4.70 –0.04 树皮 Bark 0.619 –0.06 14.69 4.23 0.00 树枝 Branch 0.373 1.62 53.52 5.69 0.04 树叶 Leaf 0.495 0.15 6.57 4.16 0.02 人工林
Planted
forest干材 Stem wood 0.614 0.48 50.07 4.57 0.01 树皮 Bark 0.657 0.05 9.70 4.46 0.00 树枝 Branch 0.519 –1.54 50.61 7.43 –0.05 树叶 Leaf 0.577 –0.16 6.02 6.18 –0.03 枫香
Liquidambar formosana天然林
Natural
forest干材 Stem wood 0.696 –5.63 69.98 3.05 –0.07 树皮 Bark 0.866 –0.38 7.84 2.02 –0.03 树枝 Branch 0.706 –1.31 22.03 3.01 –0.05 树叶 Leaf 0.544 –0.45 6.33 5.38 –0.10 人工林
Planted
forest干材 Stem wood 0.647 0.04 90.75 6.73 0.00 树皮 Bark 0.768 0.20 10.96 5.49 0.02 树枝 Branch 0.567 –1.83 64.43 10.65 –0.05 树叶 Leaf 0.767 –0.38 5.66 6.48 –0.07 表 6 各组分生物量生长模型的权函数估计结果
Table 6 Estimates of weight functions for biomass growth models of different components
树种 Species 起源 Origin 各组分权函数 Weight function estimate of each component 干材 Stem wood 树皮 Bark 树枝 Branch 树叶 Leaf 樟树 Cinnamomum
camphora天然林 Natural forest g(T)=1/T 0.829 2 g(T)=1/T 3.235 6 g(T)=1/T 1.776 4 g(T)=1/T 1.787 9 人工林 Planted forest g(T)=1/T 1.414 5 g(T)=1/T 2.579 3 g(T)=1/T 2.184 0 g(T)=1/T 2.437 1 木荷 Schima
superba天然林 Natural forest g(T)=1/T 1.941 8 g(T)=1/T 1.828 5 g(T)=1/T 0.977 5 g(T)=1/T 0.946 4 人工林 Planted forest g(T)=1/T 4.029 9 g(T)=1/T 4.262 4 g(T)=1/T 5.148 7 g(T)=1/T 2.708 0 枫香 Liquidambar
formosana天然林 Natural forest g(T)=1/T 1.030 5 g(T)=1/T 1.444 1 g(T)=1/T 1.219 0 g(T)=1/T 2.007 0 人工林 Planted forest g(T)=1/T 0.608 1 g(T)=1/T 0.637 6 g(T)=1/T 1.057 4 g(T)=1/T 0.847 3 -
[1] DIXON R K, SOLOMON A M, BROWN S, et al. Carbon pools and flux of global forest ecosystems[J]. Science, 1994, 263(5144): 185-190. doi: 10.1126/science.263.5144.185
[2] GOODALE C, APPS M, BIRDSEY R, et al. Forest carbon sinks in the Northern Hemisphere[J]. Ecol Appl, 2002, 12(3): 891-899. doi: 10.1890/1051-0761(2002)012[0891:FCSITN]2.0.CO;2
[3] 董利虎, 李凤日, 贾炜玮, 等. 含度量误差的黑龙江省主要树种生物量相容性模型[J]. 应用生态学报, 2011, 22(10): 2653-2661. [4] VOGT K. Carbon budgets of temperate forest ecosystems[J]. Tree Physiol, 1991, 9(1/2): 68-86.
[5] KURZ W A, BEUKEMA S J, APPS M J. Estimation of root biomass and dynamics for the carbon budget model of the Canadian forest sector[J]. For Res, 1996, 26(11): 1973-1979.
[6] BROWN S. Measuring carbon in forests: Current status and future challenges[J]. Environ Pollut, 2002, 116(3): 363-372. doi: 10.1016/S0269-7491(01)00212-3
[7] SATOO T, MADGWICK H A I. Forest Biomass[M]. Boston: Martinus Nijhoff/Dr. W. Junk Publishers, 1982: 1-152.
[8] WANG J R, LETCHFORD T, COMEAU P, et al. Above- and below-ground biomass and nutrient distribution of a paper birch and subalpine fir mixed-species stand in the Sub-Boreal Spruce zone of British Columbia[J]. For Ecol Manag, 2000, 130(1/2/3): 17-26.
[9] LITTON C M, RYAN M G, TINKER D B, et al. Belowground and aboveground biomass in young post fire lodge pole pine forests of contrasting tree density[J]. For Res, 2003, 33(2): 351-363.
[10] TATENO R, HISHI T, TAKEDA H. Above- and belowground biomass and net primary production in a cool-temperate deciduous forest in relation to topographical changes in soil nitrogen[J]. For Ecol Manag, 2004, 1936(3): 297-306.
[11] 赵菡, 雷渊才, 符利勇. 江西省不同立地等级的马尾松林生物量估计和不确定性度量[J]. 林业科学, 2017, 53(8): 81-93. [12] HELMISAARI H S, MAKKONEN K, KELLOMAKI S, et al. Below- and above-ground biomass, production and nitrogen use in Scots pine stands in eastern Finland[J]. For Ecol Manag, 2002, 165(1/2/3): 317-326.
[13] SPRIZZA L. Age-related equations for above- and below- ground biomass of a Eucalyptus hybrid in Congo[J]. For Ecol Manag, 2005, 205(1): 199-214.
[14] 施文涛, 谢昕云, 刘西军, 等. 安徽大别山区杉木人工林乔木层生物量模型及碳贮量[J]. 长江流域资源与环境, 2015, 24(5): 758-764. [15] 刘茂秀, 史军辉, 王新英, 等. 塔河流域天然胡杨林不同林龄地上生物量及碳储量[J]. 水土保持通报, 2016, 36(5): 326-332. [16] PEICHL M, ARAIN M A. Allometry and partitioning of above- and belowground tree biomass in an age-sequence of white pine forests[J]. For Ecol Manag, 2007, 253(1): 68-80.
[17] WARING R H, RUNNING S W. Forest ecosystems analysis at multiple scales[M]. 2nd ed. San Diego, California: Academic Press, 1998: 1-431.
[18] 符利勇, 雷渊才, 孙伟, 等. 不同林分起源的相容性生物量模型构建[J]. 生态学报, 2014, 34(6): 1461-1470. [19] WANG C. Biomass allometric equations for 10 co-occurring tree species in Chinese temperate forests[J]. For Ecol Manag, 2006, 222(1): 9-16.
[20] CAIMS M A, BROWN S, HELMER E H, et al. Root biomass allocation in the world’s upland forests[J]. Oecologia, 1997, 111(1): 1-11. doi: 10.1007/s004420050201
[21] 韩畅, 宋敏, 杜虎, 等. 广西不同林龄杉木、马尾松人工林根系生物量及碳储量特征[J]. 生态学报, 2017, 37(7): 2282-2289. [22] 曾伟生, 唐守正. 利用度量误差模型方法建立相容性立木生物量方程系统[J]. 林业科学研究, 2010, 23(6): 797-802. [23] 曾伟生, 王雪军, 陈振雄, 等. 林分起源对立木生物量模型的影响分析[J]. 林业资源管理, 2014(2): 40-45. [24] 刘四海, 曾伟生. 马尾松宏观尺度单木生长模型研究[J]. 林业资源管理, 2017(2): 28-33. [25] ZAVITKOVSKI J. Dry weight and leaf area of aspen trees in the northern Wisconsin[C]// Proceedings of the XV IUFRO Congress on Forest Biomass Studies, IUFRO on Forest Biomass Studies, Section 25, Growth and Yield, March 15-20, University of Florida, Gainsville, Florida, USA, 1971: 191-205.





 下载:
下载:
